Does mathematics prove God?

Mathematics is not like science

The scientific method is fairly simple. When faced with a question, work out a way to test possible answers, run the test and learn from the result. It’s very real world based. If the world is different to what we expected, we get an unexpected answer. Scientific “facts” are always somewhat provisional, that is, new information or deeper understandings may require apparent facts to be reconsidered. We can say that the sciences are empirical. In a different universe, science would be different.

But mathematics is very different. Real world experimentation generally isn’t needed or appropriate for basic mathematical truths, such as Pythagorus’ theorem about right angle triangles. They are established by proofs. They are not empirical, but necessarily true. They would be true in all universes, not just our one. (Of course, when mathematics is used by physicists, the equations are empirical.)

Science depends on mathematics

In most of the sciences, especially physics, mathematics forms an important part of the scientific method. Equations may be developed to explain the data, or probability may be used to define how precise an answer can be known. The laws of physics are expressed in mathematical terms.

As Galileo once wrote: “The book of nature is written in the language of mathematics.” In fact mathematics is often used to predict physical facts that are later shown to be true. For example, gravitational equations were used to predict the existence of the planet Neptune and the dwarf planet Pluto.

Scientists generally believe that the laws of physics and the mathematical equations that describe them are the same right throughout the universe. This remarkable fact suggests that mathematics is very central to the natural world, even though it is very different to science.

Mathematics is abstract, non-physical

Modern science is carried out with the assumption of naturalism. That is, it is assumed that the natural world is a closed system and natural laws always hold. Many scientists are naturalists – they believe not only that the natural world is a closed system, but there is nothing else “outside” it. Theists of course believe there is a God “outside” the natural world, but scientists who are christians still do their science assuming natural laws are applying. If God intervenes, it is in ways that don’t nullify the natural laws.

But if the physical world is all there is, how do we explain mathematics? Mathematical equations are non-physical, abstract yet they are undeniably powerful in that they describe the natural world and how it operates.

Sometimes, entire fields of theoretical mathematics are developed with no physical reality or application in mind, and then later the field is found to be relevent to some aspect of the physical world. For example, Bernhard Riemann described non-Euclidean geometries, then more than half a century later Einstein used those geometries to build his general theory of relativity.

Even stranger, some aspects of mathematics cannot describe anything real, yet they are still useful in the real world. An example is imaginery numbers (see box). So an abstract non-physical something that can’t actually exist helps describe the world.

Discovered or invented?

So is mathematics something human beings have invented, like language or culture, or is it something inherent in the universe that we are in the process of discovering?

An invention?

One view is that humans have invented mathematics for just this task of describing the physical universe. As the human race has gained knowledge, our ability to understand and describe the physical universe using mathematics has increased. So mathematics works because humans are clever.

Prof James Brown: “Mathematics is a game. It’s something that we have created.”

Proponents of this view point to the fact that many of our mathematical equations only describe the universe approximately, and could be improved. Thus, they say, it is clearly a human artefact.

Discovered?

But other scientists argue that humans didn’t invent mathematics. The basics of mathematics is already “there”.

Physicist Paul Davies: “Scientists do not use mathematics merely as a convenient way of organising the data. They believe that mathematical relationships reflect real aspects of the physical world. Science relies on the assumption that we live in an ordered Universe that is subject to precise mathematical laws.”

Proponents of this view argue that we cannot make 1 + 1 = 3, nor change Pythagorus’ Theorem. So mathematics can’t be an invention.

The essence?

Cosmologist Max Tegmark has gone one step further and argued that the universe is mathematical in nature; it is a mathematical structure. “Mathematical existence equals physical existence, and all structures that exist mathematically exist physically as well.”

I don’t pretend to fully understand what Tegmark means, but it certainly suggests that mathematics is discovered not invented, because the universe is discovered by us, and not invented.

Mathematics is finding patterns

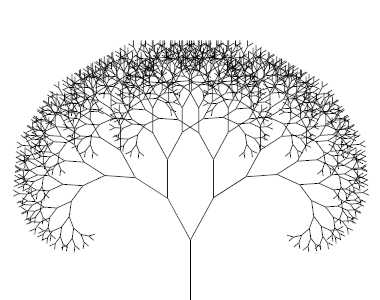
We recognise that mathematics is a language for describing patterns in the world. The path of a ball thrown into the air is described by a simple equation. Very simple equations describe the electrical currents running through my computer as I type this.

Even when a simple equation cannot be found, mathematics can help. Fractals are complex shapes that cannot be described by simple equations, but can be produced by applying a simple mathematical procedure over and over again.

This suggests that the symbols and terms, and many of the applications of mathematics are invented, but the core insights of mathematics are discovered truths that would are true regardless of our universe.

It seems mathematics is both discovered and invented.

So we essentially have two questions:

1. Why is the physical world so consistent in the way it “works”? Why are there universal laws of nature?
2. How is it that the physical world can be described by mathematical equations?

Mystery or miracle?

A mystery?

If, as seems to be the case, mathematics is built into the structure of the universe, why is this the case?

There seems to be no reason why this is inevitable. Chaotic non-mathematical universes can be imagined and seem possible. So perhaps it is just a fact, a mystery, the way things are. Perhaps it could have been different, but it’s not.

Nobel laureate physicist Eugene Wigner wrote “the enormous usefulness of mathematics in the natural sciences is something bordering on the mysterious and that there is no rational explanation for it. …. The miracle of the appropriateness of the language of mathematics for the formulation of the laws of physics is a wonderful gift which we neither understand nor deserve.”.

A miracle?

The fortuitous and almost miraculous nature of the effectiveness of mathematics has led some to argue that it is another sign of a designer. Philosopher William Lane Craig suggests that mathematics works so well because God designed the universe on a mathematical model and then built it with the physics of the universe. It’s like building a house – mathematics is like the architect’s plan and the physics of the universe is like the building.

Craig suggests that this understanding is the basis for a theistic argument. If there’s no God,the applicability of mathematics is a happy coincidence. But it appears it isn’t just a coincidence, so God must exist.

Proving God by mathematics?

Kurt Gödel was an eminent mathmatician who lived last century. He is famous for proving mathematically that all mathematical systems are incomplete and that every mathematical system will have some statements that can never be proven.

He also developed a so-called mathematical proof for the existence of God, based on the Ontological argument for God’s existence (see box). Gödel’s argument has 12 propositions – 5 axioms, 3 definitions and 4 theorems or conclusions – all expressed in symbolic logic (which to my mind is philosophical, not mathematical).

The final conclusion is: “It is necessary that God exists.”

The argument has been criticised, of course (particularly some of the axioms or premises), defended and refined. In particular, it is argued that if the axioms are assumed true, other conclusions can be drawn that show, reductio ad absurdum, that the axioms cannot all be true.

Computer Scientists ‘Prove’ God Exists

That’s what the headlines said, but it was of course not quite that simple. Two German computer scientists used “higher-order automated reasoning techniques” which utilise natural language processing (see box) to analyse a slightly modified form of Gödel’s argument.

They found that many of the objections to the argument were valid, but if the axioms are accepted the existence of God is proved.

Few people see this as a step forward in theistic argumentation – it was intended rather to demonstrate the use of computers to resolve complex logical arguments. Nevertheless, the conclusion is interesting.

So, does mathematics prove God exists?

Neither the mystery of why the universe is so inexorably mathematical, nor the “proof” of Gödel’s argument, are likely to be compelling reasons for an atheist to convert.

Nevertheless, they add some weight to the many arguments and reasons to think God’s existence is the best expanation of many different aspects of the universe.

Main photo: mashup by unkleE from originals of Albert Einstein (by Ferdinand Schmutzer) and Einstein’s blackboard (by decltype on Wikimedia Commons). Fractal diagrams from Wikipedia.

You may also like these

• 
  A challenge to materialism?
• 
  God and time
• 
  Is God a good theory?
• 
  A universe out of nothing vs God out of nowhere
• 
  An alternative fine-tuning argument

Feedback on this page

Comment on this topic or leave a note on the Guest book to let me know you’ve visited.